Modulus is the absolute value of the expression. To at least somehow designate a module, it is customary to use straight brackets. The value that is enclosed in even brackets is the value that is taken modulo. The process of solving any module consists in opening those same direct brackets, which are called modular brackets in mathematical language. Their disclosure occurs according to a certain number of rules. Also, in the order of solving modules, there are also sets of values ​​of those expressions that were in module brackets. In most cases, the module is expanded in such a way that the expression that was submodule gets both positive and negative values, including the value zero. If you deviate from set properties module, then in the process various equations or inequalities from the original expression are compiled, which then need to be solved. Let's figure out how to solve modules.

Solution Process

The solution of the module begins with writing the original equation with the module. To answer the question of how to solve equations with a modulus, you need to open it completely. To solve such an equation, the module is expanded. All modular expressions must be considered. It is necessary to determine at what values ​​of the unknown quantities included in its composition, the modular expression in brackets vanishes. In order to do this, it is enough to equate the expression in modular brackets to zero, and then calculate the solution of the resulting equation. The values ​​found must be recorded. In the same way, you also need to determine the value of all unknown variables for all modules in this equation. Next, it is necessary to deal with the definition and consideration of all cases of the existence of variables in expressions when they are different from the value zero. To do this, you need to write down some system of inequalities corresponding to all modules in the original inequality. The inequalities must be drawn up so that they cover all the available and possible values ​​for the variable that are found on the number line. Then you need to draw for visualization this same number line, on which to put all the obtained values ​​in the future.

Almost everything can now be done online. The module is no exception to the rules. You can solve it online on one of the many modern resources. All those values ​​of the variable that are in the zero module will be a special constraint that will be used in the solution process modular equation. In the original equation, it is required to expand all available modular brackets, while changing the sign of the expression so that the values ​​of the desired variable coincide with those values ​​that are visible on the number line. The resulting equation must be solved. The value of the variable, which will be obtained in the course of solving the equation, must be checked against the restriction that is set by the module itself. If the value of the variable fully satisfies the condition, then it is correct. All roots that will be obtained in the course of solving the equation, but will not fit the constraints, must be discarded.

We don't choose math her profession, and she chooses us.

Russian mathematician Yu.I. Manin

Modulo Equations

The most difficult problems to solve in school mathematics are equations containing variables under the module sign. To successfully solve such equations, it is necessary to know the definition and basic properties of the module. Naturally, students should have the skills to solve equations of this type.

Basic concepts and properties

Modulus (absolute value) of a real number denoted and is defined as follows:

TO simple properties module include the following relations:

Note, that the last two properties hold for any even degree.

Also, if , where , then and

More complex module properties, which can be effectively used in solving equations with modules, are formulated by means of the following theorems:

Theorem 1.For any analytic functions and the inequality

Theorem 2. Equality is the same as inequality.

Theorem 3. Equality is equivalent to the inequality.

Consider typical examples of solving problems on the topic “Equations, containing variables under the module sign.

Solving Equations with Modulus

The most common method in school mathematics for solving equations with a modulus is the method, based on module expansion. This method is generic, however, in the general case, its application can lead to very cumbersome calculations. In this regard, students should also be aware of other, more effective methods and methods for solving such equations. In particular, need to have the skills to apply theorems, given in this article.

Example 1 Solve the equation. (one)

Solution. Equation (1) will be solved by the "classical" method - the module expansion method. To do this, we break the numerical axis dots and intervals and consider three cases.

1. If , then , , , and equation (1) takes the form . It follows from here. However, here , so the found value is not the root of equation (1).

2. If , then from equation (1) we obtain or .

Since then the root of equation (1).

3. If , then equation (1) takes the form or . Note that .

Answer: , .

When solving the following equations with a module, we will actively use the properties of modules in order to increase the efficiency of solving such equations.

Example 2 solve the equation.

Solution. Since and then it follows from the equation. In this regard, , , and the equation becomes. From here we get. But , so the original equation has no roots.

Answer: no roots.

Example 3 solve the equation.

Solution. Since , then . If , then , and the equation becomes.

From here we get .

Example 4 solve the equation.

Solution.Let us rewrite the equation in an equivalent form. (2)

The resulting equation belongs to equations of the type .

Taking into account Theorem 2, we can state that equation (2) is equivalent to the inequality . From here we get .

Answer: .

Example 5 Solve the equation.

Solution. This equation has the form. So , according to Theorem 3, here we have the inequality or .

Example 6 solve the equation.

Solution. Let's assume that . Because , then the given equation takes the form of a quadratic equation, (3)

where . Since equation (3) has a single positive root and , then . From here we get two roots of the original equation: and .

Example 7 solve the equation. (4)

Solution. Since the equationis equivalent to the combination of two equations: and , then when solving equation (4) it is necessary to consider two cases.

1. If , then or .

From here we get , and .

2. If , then or .

Since , then .

Answer: , , , .

Example 8solve the equation . (5)

Solution. Since and , then . From here and from Eq. (5) it follows that and , i.e. here we have a system of equations

However, this system of equations is inconsistent.

Answer: no roots.

Example 9 solve the equation. (6)

Solution. If we designate and from equation (6) we obtain

Or . (7)

Since equation (7) has the form , this equation is equivalent to the inequality . From here we get . Since , then or .

Answer: .

Example 10solve the equation. (8)

Solution.According to Theorem 1, we can write

(9)

Taking into account equation (8), we conclude that both inequalities (9) turn into equalities, i.e. there is a system of equations

However, by Theorem 3, the above system of equations is equivalent to the system of inequalities

(10)

Solving the system of inequalities (10) we obtain . Since the system of inequalities (10) is equivalent to equation (8), the original equation has a single root .

Answer: .

Example 11. solve the equation. (11)

Solution. Let and , then the equation (11) implies the equality .

From this it follows that and . Thus, here we have a system of inequalities

The solution to this system of inequalities are and .

Answer: , .

Example 12.solve the equation. (12)

Solution. Equation (12) will be solved by the method of successive expansion of modules. To do this, consider several cases.

1. If , then .

1.1. If , then and , .

1.2. If , then . But , therefore, in this case, equation (12) has no roots.

2. If , then .

2.1. If , then and , .

2.2. If , then and .

Answer: , , , , .

Example 13solve the equation. (13)

Solution. Since the left side of equation (13) is non-negative, then and . In this regard, , and equation (13)

takes the form or .

It is known that the equation is equivalent to the combination of two equations and , solving which we get, . Because , then equation (13) has one root.

Answer: .

Example 14 Solve a system of equations (14)

Solution. Since and , then and . Therefore, from the system of equations (14) we obtain four systems of equations:

The roots of the above systems of equations are the roots of the system of equations (14).

Answer: ,, , , , , , .

Example 15 Solve a system of equations (15)

Solution. Since , then . In this regard, from the system of equations (15) we obtain two systems of equations

The roots of the first system of equations are and , and from the second system of equations we obtain and .

Answer: , , , .

Example 16 Solve a system of equations (16)

Solution. It follows from the first equation of system (16) that .

Since then . Consider the second equation of the system. Insofar as, then , and the equation becomes, , or .

If we substitute the valueinto the first equation of system (16), then , or .

Answer: , .

For a deeper study of problem solving methods, related to the solution of equations, containing variables under the module sign, you can advise tutorials from the list of recommended literature.

1. Collection of tasks in mathematics for applicants to technical universities / Ed. M.I. Scanavi. - M .: World and Education, 2013. - 608 p.

2. Suprun V.P. Mathematics for high school students: tasks increased complexity. - M .: KD "Librocom" / URSS, 2017. - 200 p.

3. Suprun V.P. Mathematics for high school students: non-standard methods for solving problems. - M .: KD "Librocom" / URSS, 2017. - 296 p.

Do you have any questions?

To get the help of a tutor - register.

site, with full or partial copying of the material, a link to the source is required.

One of the most difficult topics for students is solving equations containing a variable under the modulus sign. Let's see for a start what is it connected with? Why, for example, most children click quadratic equations like nuts, but with such complex concept how does the module have so many problems?

In my opinion, all these difficulties are associated with the lack of clearly formulated rules for solving equations with a modulus. So, when solving a quadratic equation, the student knows for sure that he needs to first apply the discriminant formula, and then the formulas for the roots of the quadratic equation. But what if a module is encountered in the equation? We will try to clearly describe the necessary plan of action in the case when the equation contains an unknown under the modulus sign. We give several examples for each case.

But first, let's remember module definition. So, the modulus of the number a the number itself is called if a non-negative and -a if the number a less than zero. You can write it like this:

|a| = a if a ≥ 0 and |a| = -a if a< 0

Speaking about the geometric meaning of the module, it should be remembered that each real number corresponds to a certain point on the number axis - its to coordinate. So, the module or the absolute value of a number is the distance from this point to the origin of the numerical axis. The distance is always given as a positive number. Thus, the modulus of any negative number is a positive number. By the way, even at this stage, many students begin to get confused. Any number can be in the module, but the result of applying the module is always a positive number.

Now let's move on to solving the equations.

1. Consider an equation of the form |x| = c, where c is a real number. This equation can be solved using the definition of the modulus.

We divide all real numbers into three groups: those that are greater than zero, those that are less than zero, and the third group is the number 0. We write the solution in the form of a diagram:

(±c if c > 0

If |x| = c, then x = (0 if c = 0

(no roots if with< 0

1) |x| = 5, because 5 > 0, then x = ±5;

2) |x| = -5, because -5< 0, то уравнение не имеет корней;

3) |x| = 0, then x = 0.

2. An equation of the form |f(x)| = b, where b > 0. To solve this equation, it is necessary to get rid of the modulus. We do it like this: f(x) = b or f(x) = -b. Now it is necessary to solve separately each of the obtained equations. If in the original equation b< 0, решений не будет.

1) |x + 2| = 4, because 4 > 0, then

x + 2 = 4 or x + 2 = -4

2) |x 2 – 5| = 11, because 11 > 0, then

x 2 - 5 = 11 or x 2 - 5 = -11

x 2 = 16 x 2 = -6

x = ± 4 no roots

3) |x 2 – 5x| = -8 , because -eight< 0, то уравнение не имеет корней.

3. An equation of the form |f(x)| = g(x). According to the meaning of the module, such an equation will have solutions if its right side is greater than or equal to zero, i.e. g(x) ≥ 0. Then we have:

f(x) = g(x) or f(x) = -g(x).

1) |2x – 1| = 5x - 10. This equation will have roots if 5x - 10 ≥ 0. This is where the solution of such equations begins.

1. O.D.Z. 5x – 10 ≥ 0

2. Solution:

2x - 1 = 5x - 10 or 2x - 1 = -(5x - 10)

3. Combine O.D.Z. and the solution, we get:

The root x \u003d 11/7 does not fit according to O.D.Z., it is less than 2, and x \u003d 3 satisfies this condition.

Answer: x = 3

2) |x – 1| \u003d 1 - x 2.

1. O.D.Z. 1 - x 2 ≥ 0. Let's solve this inequality using the interval method:

(1 – x)(1 + x) ≥ 0

2. Solution:

x - 1 \u003d 1 - x 2 or x - 1 \u003d - (1 - x 2)

x 2 + x - 2 = 0 x 2 - x = 0

x = -2 or x = 1 x = 0 or x = 1

3. Combine solution and O.D.Z.:

Only the roots x = 1 and x = 0 are suitable.

Answer: x = 0, x = 1.

4. An equation of the form |f(x)| = |g(x)|. Such an equation is equivalent to the following two equations f(x) = g(x) or f(x) = -g(x).

1) |x 2 - 5x + 7| = |2x – 5|. This equation is equivalent to the following two:

x 2 - 5x + 7 = 2x - 5 or x 2 - 5x +7 = -2x + 5

x 2 - 7x + 12 = 0 x 2 - 3x + 2 = 0

x = 3 or x = 4 x = 2 or x = 1

Answer: x = 1, x = 2, x = 3, x = 4.

5. Equations solved by the substitution method (change of variable). This method solutions are easiest to explain in specific example. So, let a quadratic equation with a modulus be given:

x 2 – 6|x| + 5 = 0. By the property of the module x 2 = |x| 2 , so the equation can be rewritten as follows:

|x| 2–6|x| + 5 = 0. Let's make the change |x| = t ≥ 0, then we will have:

t 2 - 6t + 5 \u003d 0. Solving this equation, we get that t \u003d 1 or t \u003d 5. Let's return to the replacement:

|x| = 1 or |x| = 5

x = ±1 x = ±5

Answer: x = -5, x = -1, x = 1, x = 5.

Let's look at another example:

x 2 + |x| – 2 = 0. By the property of the module x 2 = |x| 2 , so

|x| 2 + |x| – 2 = 0. Let's make the change |x| = t ≥ 0, then:

t 2 + t - 2 \u003d 0. Solving this equation, we get, t \u003d -2 or t \u003d 1. Let's return to the replacement:

|x| = -2 or |x| = 1

No roots x = ± 1

Answer: x = -1, x = 1.

6. Another type of equations is equations with a "complex" modulus. Such equations include equations that have "modules within a module". Equations of this type can be solved using the properties of the module.

1) |3 – |x|| = 4. We will act in the same way as in equations of the second type. Because 4 > 0, then we get two equations:

3 – |x| = 4 or 3 – |x| = -4.

Now let's express the module x in each equation, then |x| = -1 or |x| = 7.

We solve each of the resulting equations. There are no roots in the first equation, because -one< 0, а во втором x = ±7.

Answer x = -7, x = 7.

2) |3 + |x + 1|| = 5. We solve this equation in a similar way:

3 + |x + 1| = 5 or 3 + |x + 1| = -5

|x + 1| = 2 |x + 1| = -8

x + 1 = 2 or x + 1 = -2. There are no roots.

Answer: x = -3, x = 1.

There is also a universal method for solving equations with a modulus. This is the spacing method. But we will consider it further.

site, with full or partial copying of the material, a link to the source is required.

MBOU secondary school №17 Ivanov

« Modulo Equations»
Methodical development

Compiled

math teacher

Lebedeva N.V.

20010

Explanatory note

Chapter 1 Introduction

Section 2. Main features Section 3. Geometric interpretation of the concept of the modulus of a number Section 4. Graph of the function y = |x| Section 5 Conventions

Chapter 2

Section 1. Equations of the form |F(х)| = m (protozoa) Section 2. Equations of the form F(|х|) = m Section 3. Equations of the form |F(х)| = G(x) Section 4. Equations of the form |F(х)| = ± F(x) (beautiful) Section 5. Equations of the form |F(х)| = |G(x)| Section 6. Examples of solving non-standard equations Section 7. Equations of the form |F(х)| + |G(x)| = 0 Section 8. Equations of the form |а 1 x ± в 1 | ± |a 2 x ± in 2 | ± …|a n x ± in n | = m Section 9. Equations Containing Multiple Modules

Chapter 3. Examples of solving various equations with a modulus.

Section 1. Trigonometric Equations Section 2 exponential equations Section 3 Logarithmic Equations Section 4. Irrational Equations Section 5. Tasks of advanced complexity Answers to the exercises Bibliography

Explanatory note.

The concept of the absolute value (modulus) of a real number is one of its essential characteristics. This concept is widely used in various branches of physical, mathematical and technical sciences. In the practice of teaching a mathematics course in secondary school in accordance with the Program of the Ministry of Defense of the Russian Federation, the concept of “absolute value of a number” is encountered repeatedly: in the 6th grade, the definition of a module, its geometric meaning, is introduced; in the 8th grade, the concept of absolute error is formed, the solution of the simplest equations and inequalities containing the modulus is considered, the properties of the arithmetic square root; in the 11th grade, the concept is found in the section “Root nth degree." Teaching experience shows that students often encounter difficulties in solving tasks that require knowledge of this material, and often skip before starting to complete. In the texts of examination tasks for the course of the 9th and 11th grades, similar tasks are also included. In addition, the requirements that universities impose on school graduates differ, namely, more high level than the requirements of the school curriculum. For life in modern society very important is the formation of a mathematical style of thinking, manifested in certain mental skills. In the process of solving problems with modules, the ability to apply such techniques as generalization and concretization, analysis, classification and systematization, analogy is required. The solution of such tasks allows you to check the knowledge of the main sections of the school course, the level logical thinking, initial research skills. this work is devoted to one of the sections - the solution of equations containing the module. It consists of three chapters. The first chapter introduces the basic concepts and the most important theoretical calculations. The second chapter proposes nine basic types of equations containing the module, considers methods for solving them, and analyzes examples of different levels of complexity. The third chapter offers more complex and non-standard equations (trigonometric, exponential, logarithmic and irrational). For each type of equations there are exercises for independent solution (answers and instructions are attached). The main purpose of this work is to provide methodological assistance to teachers in preparing for lessons and in organizing optional courses. The material can also be used as study guide for high school students. The tasks proposed in the work are interesting and not always easy to solve, which makes it possible to make the learning motivation of students more conscious, test their abilities, and improve the level of preparation of school graduates for entering universities. A differentiated selection of the proposed exercises implies a transition from the reproductive level of assimilation of the material to the creative one, as well as the opportunity to teach how to apply their knowledge in solving non-standard problems.

Chapter 1. Introduction.

Section 1. Determination of the absolute value .

Definition : The absolute value (modulus) of a real number a is called a non-negative number: a or -a. Designation: │ a │ The entry reads as follows: “module of the number a” or “absolute value of the number a”

│ a if a > 0

│a│ = │ 0 if a = 0 (1)

│ - a, if a
Examples: 1) │2,5│ = 2,5 2) │-7│ = 7 3) │1 - √2│ = √2 – 1

Expand expression module:

a) │x - 8│ if x > 12 b) │2x + 3│ if x ≤ -2 │x - 8│= x - 8 │ 2x + 3│= - 2x - 3

Section 2. Basic properties.

Consider the basic properties of the absolute value. Property #1: Opposite numbers have equal modules, i.e. │а│=│-а│ Let us show the correctness of the equality. Let's write down the definition of the number - a : │- a│= (2) Let's compare sets (1) and (2). Obviously, the definitions of the absolute values ​​of numbers a and - a match up. Hence, │а│=│-а│
When considering the following properties, we confine ourselves to their formulation, since their proof is given in Property #2: The absolute value of the sum of a finite number of real numbers does not exceed the sum of the absolute values ​​of the terms: Property #3: The absolute value of the difference between two real numbers does not exceed the sum of their absolute values: │а - в│ ≤│а│+│в│ Property #4: The absolute value of the product of a finite number of real numbers is equal to the product of the absolute values ​​of the factors: │а · в│=│а│·│в│ Property #5: The absolute value of the quotient of real numbers is equal to the quotient of their absolute values:

Section 3. Geometric interpretation of the concept of the modulus of a number.

Each real number can be associated with a point on the number line, which will be a geometric representation of this real number. Each point on the number line corresponds to its distance from the origin, i.e. the length of the segment from the origin to the given point. This distance is always considered as a non-negative value. Therefore, the length of the corresponding segment will be the geometric interpretation of the absolute value of the given real number

The presented geometric illustration clearly confirms property No. 1, i.e. moduli of opposite numbers are equal. From here, the validity of the equality is easily understood: │x - a│= │a - x│. It also becomes more obvious to solve the equation │х│= m, where m ≥ 0, namely x 1.2 = ± m. Examples: 1) │х│= 4 x 1.2 = ± 4 2) │х - 3│= 1
x 1.2 = 2; 4

Section 4. Graph of the function y \u003d │х│

The domain of this function is all real numbers.

Section 5. Symbols.

In the future, when considering examples of solving equations, the following will be used. conventions: ( - system sign [ - set sign When solving a system of equations (inequalities), the intersection of the solutions of the equations (inequalities) included in the system is found. When solving a set of equations (inequalities), a union of solutions of the equations (inequalities) included in the set is found.

Chapter 2

In this chapter, we will look at algebraic ways to solve equations containing one or more modules.

Section 1. Equations of the form │F (х) │= m

An equation of this type is called the simplest. It has a solution if and only if m ≥ 0. By the definition of the modulus, the original equation is equivalent to the combination of two equations: │ F(x)│=m
Examples:
№1. Solve the equation: │7x - 2│= 9


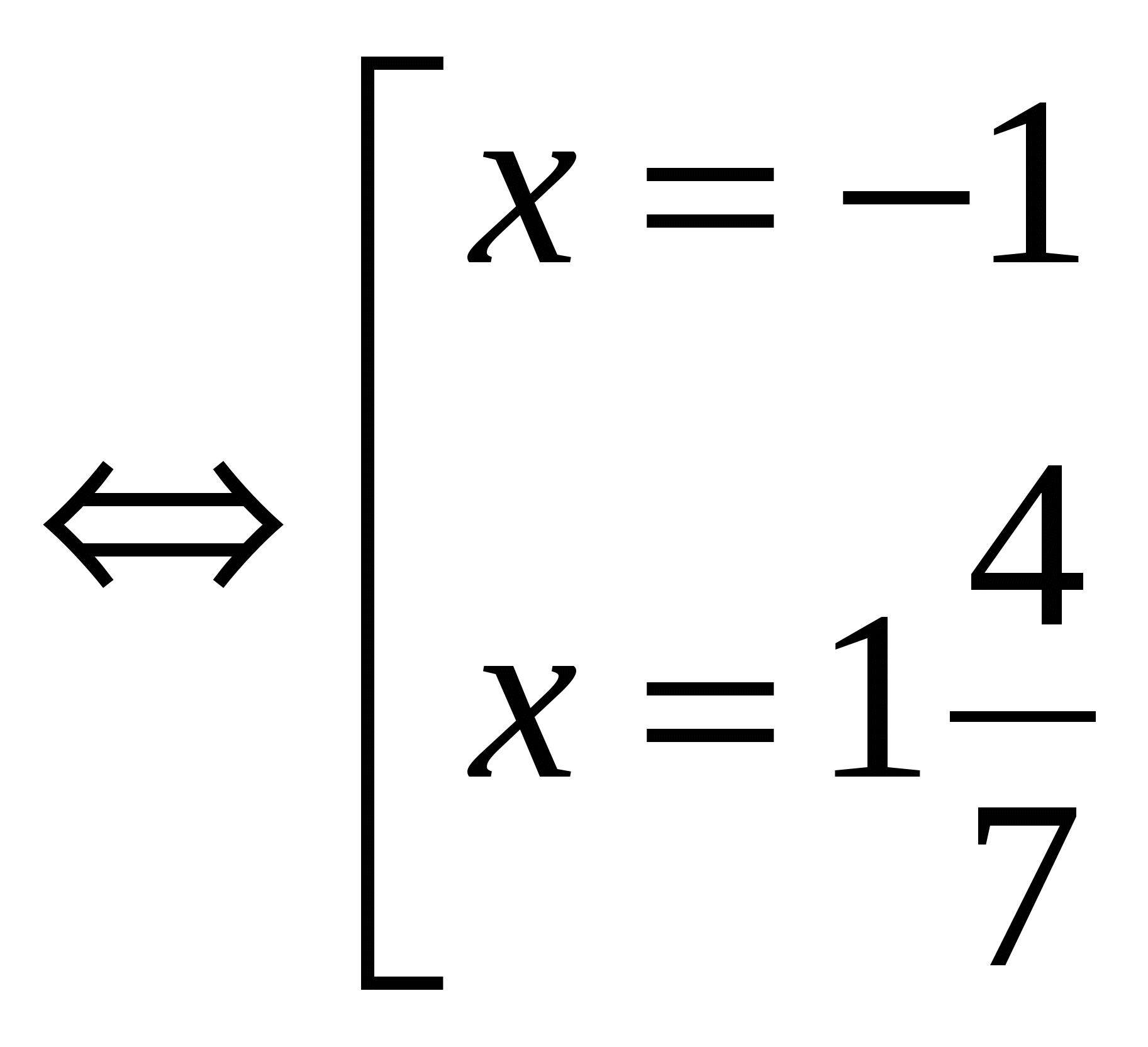Answer: x 1 = - 1; X 2 = 1 4 / 7 №2
│x 2 + 3x + 1│= 1

x 2 + 3x + 2 = 0 x 2 + 3x = 0 x 1 = -1; x 2 \u003d -2 x (x + 3) \u003d 0 x 1 \u003d 0; x 2 = -3 Answer: the sum of the roots is - 2.№3
│x 4 -5x 2 + 2│= 2 x 4 - 5x 2 = 0 x 4 - 5x 2 + 4 = 0 x 2 (x 2 - 5) = 0 denote x 2 = m, m ≥ 0 x = 0 ; ±√5 m 2 – 5m + 4 = 0 m = 1; 4 – both values ​​satisfy the condition m ≥ 0 x 2 = 1 x 2 = 4 x = ± 1 x = ± 2 Answer: the number of roots of equation 7. Exercises:
№1. Solve the equation and indicate the sum of the roots: │x - 5│= 3 №2 . Solve the equation and indicate the smaller root: │x 2 + x │ \u003d 0 №3 . Solve the equation and indicate the larger root: │x 2 - 5x + 4 │ \u003d 4 №4 .Solve the equation and indicate the whole root: │2x 2 - 7x + 6│ \u003d 1 №5 .Solve the equation and indicate the number of roots: │x 4 - 13x 2 + 50 │ = 14

Section 2. Equations of the form F(│х│) = m

The function argument on the left side is under the modulo sign, while the right side is independent of the variable. Let us consider two ways of solving equations of this type. 1 way: By definition of the absolute value, the original equation is equivalent to the totality of two systems. In each of which a condition is imposed on the submodule expression. F(│х│) =m
Since the function F(│х│) is even on the entire domain of definition, the roots of the equations F(х) = m and F(-х) = m are pairs of opposite numbers. Therefore, it suffices to solve one of the systems (when considering the examples in this way, the solution of one system will be given). 2 way: Application of the method of introducing a new variable. In this case, the designation │х│= a is introduced, where a ≥ 0. This method less voluminous in design.
Examples: №1 . Solve the equation: 3x 2 - 4│x│ = - 1 Let's use the introduction of a new variable. Denote │x│= a, where a ≥ 0. We get the equation 3a 2 - 4a + 1 = 0 D = 16 - 12 = 4 a 1 = 1 a 2 = 1 / 3 We return to the original variable: │x│ = 1 and │х│= 1/3. Each equation has two roots. Answer: x 1 = 1; X 2 = - 1; X 3 = 1 / 3 ; X 4 = - 1 / 3 . №2. Solve the equation: 5x 2 + 3│x│- 1 \u003d 1 / 2 │x│ + 3x 2
Let's find the solution of the first set system: 4x 2 + 5x - 2 \u003d 0 D \u003d 57 x 1 \u003d -5 + √57 / 8 x 2 \u003d -5-√57 / 8 Note that x 2 does not satisfy the condition x ≥ 0. By the solution the second system will be the opposite number x 1 . Answer: x 1 = -5+√57 / 8 ; X 2 = 5-√57 / 8 .№3 . Solve the equation: x 4 - │х│= 0 Denote │х│= a, where a ≥ 0. We get the equation a 4 - a \u003d 0 a (a 3 - 1) \u003d 0 a 1 \u003d 0 a 2 \u003d 1 We return to the original variable: │х│=0 and │х│= 1 x = 0; ± 1 Answer: x 1 = 0; X 2 = 1; X 3 = - 1.
Exercises: №6. Solve the equation: 2│х│ - 4.5 = 5 - 3/8 │х│ №7 . Solve the equation, in the answer indicate the number of roots: 3x 2 - 7│x│ + 2 = 0 №8 . Solve the equation, in the answer indicate the whole solutions: x 4 + │х│ - 2 = 0

Section 3. Equations of the form │F(х)│ = G(х)

The right side of an equation of this type depends on a variable and, therefore, has a solution if and only if the right side is a function G(x) ≥ 0. The original equation can be solved in two ways: 1 way: Standard, based on the disclosure of the module based on its definition and consists in an equivalent transition to the combination of two systems. │ F(x)│ =G(X)

It is rational to use this method in the case of a complex expression for the function G(x) and a less complex expression for the function F(x), since it is supposed to solve inequalities with the function F(x). 2 way: It consists in the transition to an equivalent system in which a condition is imposed on the right side. │ F(x)│= G(x)

This method is more convenient to use if the expression for the function G(x) is less complicated than for the function F(x), since the solution of the inequality G(x) ≥ 0 is assumed. In addition, in the case of several modules, this method is recommended to use the second option. Examples: №1. Solve the equation: │x + 2│= 6 -2x
(1 way) Answer: x = 1 1 / 3 №2.
│x 2 - 2x - 1 │ \u003d 2 (x + 1)
(2 way) Answer: The product of the roots is 3.
№3. Solve the equation, in the answer write the sum of the roots:
│x - 6 │ \u003d x 2 - 5x + 9

Answer: the sum of the roots is 4.
Exercises: №9. │x + 4│= - 3x №10. Solve the equation, in the answer indicate the number of solutions: │x 2 + x - 1 │ \u003d 2x - 1 №11 . Solve the equation, in the answer indicate the product of the roots: │x + 3 │ \u003d x 2 + x - 6

Section 4. Equations of the form │F(x)│= F(x) and │F(x)│= - F(x)

Equations of this type are sometimes called "beautiful". Since the right side of the equations depends on the variable, solutions exist if and only if the right side is non-negative. Therefore, the original equations are equivalent to the inequalities:
│F(x)│= F(x) F(x) ≥ 0 and │F(x)│= - F(x) F(x) Examples: №1 . Solve the equation, in the answer indicate the smaller integer root: │5x - 3│ \u003d 5x - 3 5x - 3 ≥ 0 5x ≥ 3 x ≥ 0.6 Answer: x = 1№2. Solve the equation, in the answer indicate the length of the gap: │x 2 - 9 │ \u003d 9 - x 2 x 2 - 9 ≤ 0 (x - 3) (x + 3) ≤ 0 [- 3; 3] Answer: the length of the gap is 6.№3 . Solve the equation, in the answer indicate the number of integer solutions: │2 + x - x 2 │ = 2 + x - x 2 2 + x - x 2 ≥ 0 x 2 - x - 2 ≤ 0 [- 1; 2] Answer: 4 whole solutions.№4 . Solve the equation, in the answer indicate the largest root:
│4 - x -
│= 4 – x –
x 2 - 5x + 5 \u003d 0 D \u003d 5 x 1.2 \u003d
≈ 1,4

Answer: x = 3.

Exercises: №12. Solve the equation, in the answer indicate the whole root: │x 2 + 6x + 8 │= x 2 + 6x + 8 №13. Solve the equation, in the answer indicate the number of integer solutions: │13x - x 2 - 36│+ x 2 - 13x + 36 = 0 №14. Solve the equation, in the answer indicate an integer that is not the root of the equation:

Section 5. Equations of the form │F(x)│= │G(x)│

Since both sides of the equation are non-negative, the solution involves considering two cases: submodule expressions are equal or opposite in sign. Therefore, the original equation is equivalent to the combination of two equations: │ F(x)│= │ G(x)│
Examples: №1. Solve the equation, in the answer indicate the whole root: │x + 3│ \u003d │2x - 1│
Answer: integer root x = 4.№2. Solve the equation: │ x - x 2 - 1│ \u003d │2x - 3 - x 2 │
Answer: x = 2.№3 . Solve the equation, in the answer indicate the product of the roots:




The roots of the equation 4x 2 + 2x - 1 \u003d 0 x 1.2 \u003d - 1±√5 / 4 Answer: the product of the roots is 0.25. Exercises: №15 . Solve the equation, in the answer indicate the whole solution: │x 2 - 3x + 2│ \u003d │x 2 + 6x - 1│ №16. Solve the equation, in the answer indicate the smaller root: │5x - 3│=│7 - x│ №17 . Solve the equation, in the answer write the sum of the roots:

Section 6. Examples of solving non-standard equations

In this section, we consider examples of non-standard equations, in the solution of which the absolute value of the expression is revealed by definition. Examples:

№1. Solve the equation, in the answer indicate the sum of the roots: x │x│- 5x - 6 \u003d 0
Answer: the sum of the roots is 1 №2. . Solve the equation, in the answer indicate the smaller root: x 2 - 4x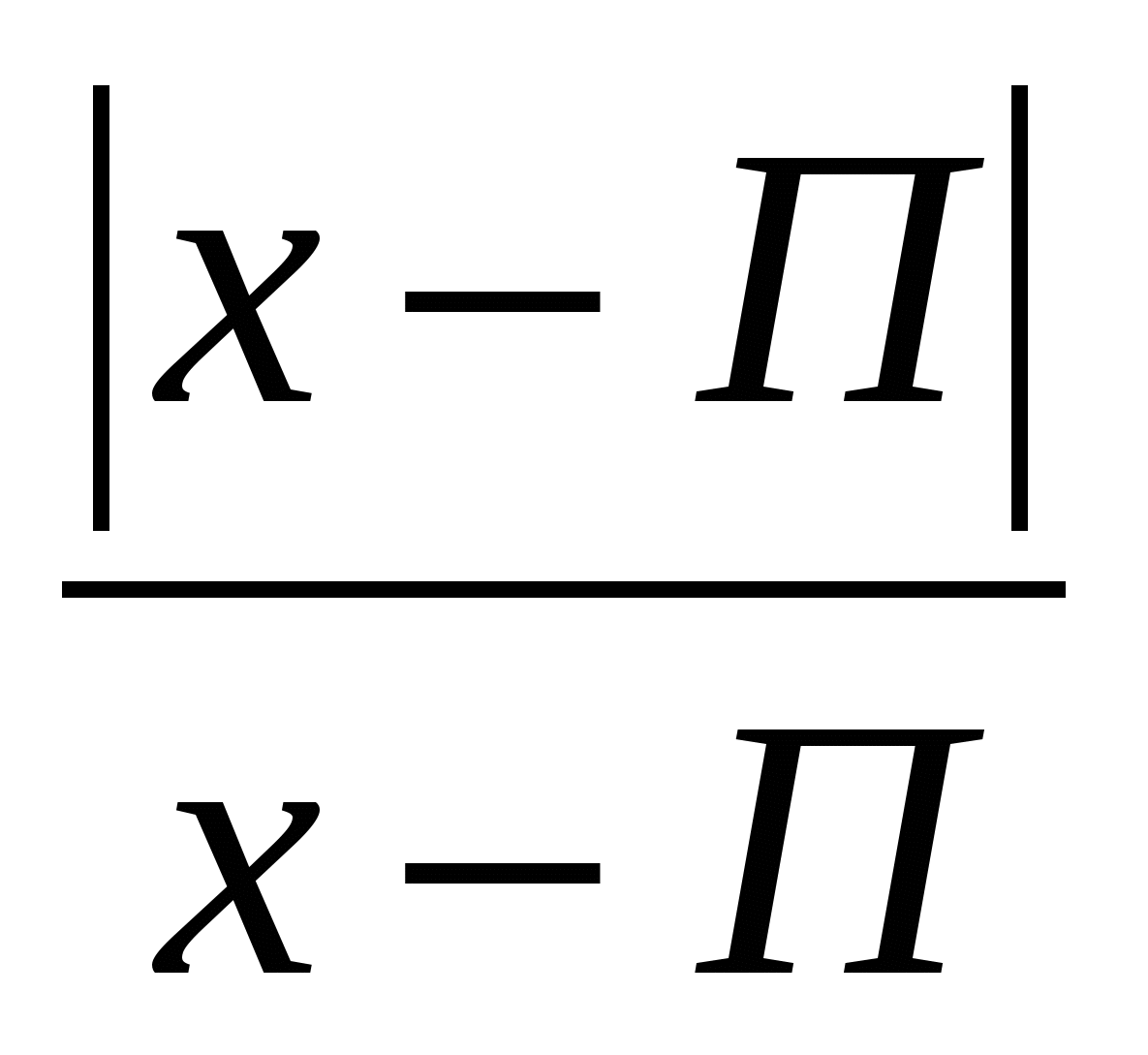
- 5 = 0
Answer: smaller root x = - 5. №3. Solve the equation:

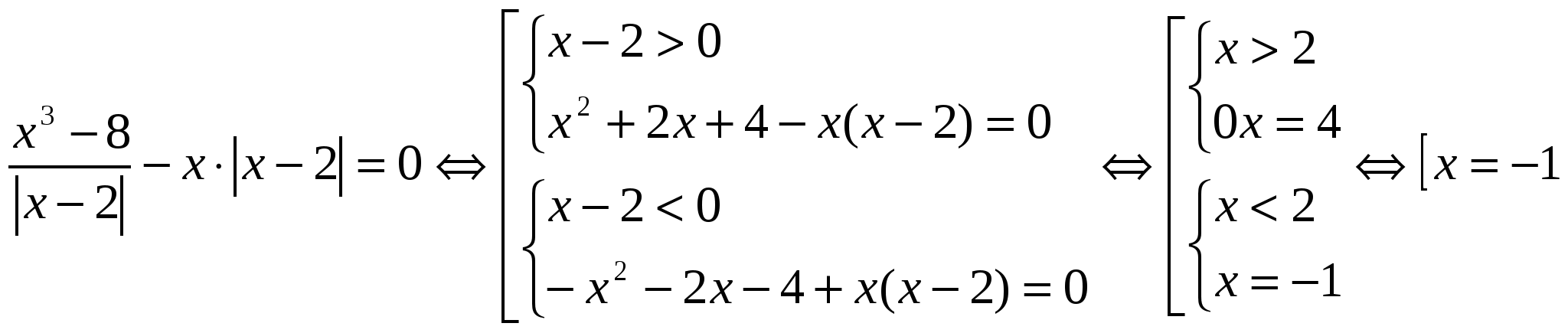Answer: x = -1. Exercises: №18. Solve the equation and write the sum of the roots: x │3x + 5│= 3x 2 + 4x + 3
№19. Solve the equation: x 2 - 3x \u003d

№20. Solve the equation:

Section 7. Equations of the form │F(x)│+│G(x)│=0

It is easy to see that on the left side of an equation of this type, the sum of non-negative quantities. Therefore, the original equation has a solution if and only if both terms are simultaneously equal to zero. The equation is equivalent to the system of equations: │ F(x)│+│ G(x)│=0
Examples: №1 . Solve the equation:
Answer: x = 2. №2. Solve the equation: Answer: x = 1. Exercises: №21. Solve the equation: №22 . Solve the equation, in the answer write the sum of the roots: №23 . Solve the equation, in the answer indicate the number of solutions:

Section 8. Equations of the form

To solve equations of this type, the method of intervals is used. If it is solved by sequential expansion of modules, then we get n sets of systems, which is very cumbersome and inconvenient. Consider the algorithm of the interval method: 1). Find Variable Values X, for which each module is equal to zero (zeros of submodule expressions):
2). The found values ​​are marked on a number line, which is divided into intervals (the number of intervals, respectively, is equal to n+1 ) 3). Determine with what sign each module is revealed at each of the obtained intervals (when making a solution, you can use a number line, marking the signs on it) 4). The original equation is equivalent to the set n+1 systems, in each of which the membership of the variable is indicated X one of the intervals. Examples: №1 . Solve the equation, in the answer indicate the largest root:
one). Let's find the zeros of submodule expressions: x = 2; x = -3 2). We mark the found values ​​on the number line and determine with what sign each module is revealed on the obtained intervals:
x – 2 x – 2 x – 2 - - + - 3 2 x 2x + 6 2x + 6 2x + 6 - + + 3)
- no solutions The equation has two roots. Answer: the largest root is x = 2. №2. Solve the equation, write the whole root in the answer:
one). Let's find the zeros of submodule expressions: x = 1.5; x = - 1 2). We mark the found values ​​on the number line and determine with what sign each module is revealed on the obtained intervals: x + 1 x + 1 x + 1 - + +
-1 1.5 х 2х – 3 2х – 3 2х – 3 - - +
3).
The last system has no solutions, therefore, the equation has two roots. When solving the equation, you should pay attention to the “-” sign in front of the second module. Answer: integer root x = 7. №3. Solve the equation, in the answer indicate the sum of the roots: 1). Let's find the zeros of submodule expressions: x = 5; x = 1; x = - 2 2). We mark the found values ​​on the number line and determine with what sign each module is revealed on the obtained intervals: x - 5 x - 5 x - 5 x - 5 - - - +
-2 1 5 x x – 1 x – 1 x – 1 x – 1 - - + + x + 2 x + 2 x + 2 x + 2 - + + +
3).
The equation has two roots x = 0 and 2. Answer: the sum of the roots is 2. №4 . Solve the equation: 1). Let's find the zeros of submodule expressions: x = 1; x = 2; x = 3. 2). Let us determine the sign with which each module is expanded on the obtained intervals. 3).
We combine the solutions of the first three systems. Answer: ; x = 5.
Exercises: №24. Solve the equation:
№25. Solve the equation, in the answer write the sum of the roots: №26. Solve the equation, in the answer indicate the smaller root: №27. Solve the equation, give the larger root in your answer:

Section 9. Equations Containing Multiple Modules

Equations containing multiple modules assume the presence of absolute values ​​in submodule expressions. The basic principle of solving equations of this type is the sequential disclosure of modules, starting with the "external". In the course of the solution, the techniques discussed in sections No. 1, No. 3 are used.

Examples: №1. Solve the equation:
Answer: x = 1; - eleven. №2. Solve the equation:
Answer: x = 0; 4; - 4. №3. Solve the equation, in the answer indicate the product of the roots: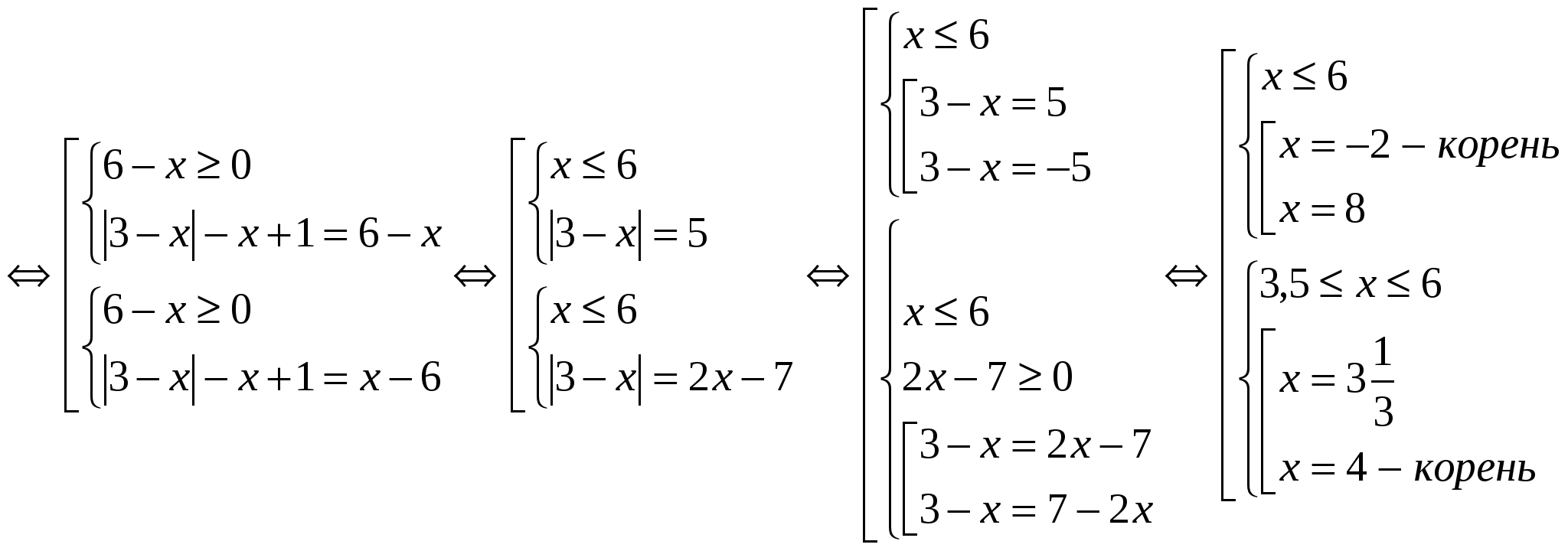
Answer: The product of the roots is 8. №4. Solve the equation:
Denote the population equations (1) and (2) and consider the solution of each of them separately for the convenience of design. Since both equations contain more than one module, it is more convenient to carry out an equivalent transition to sets of systems. (1)

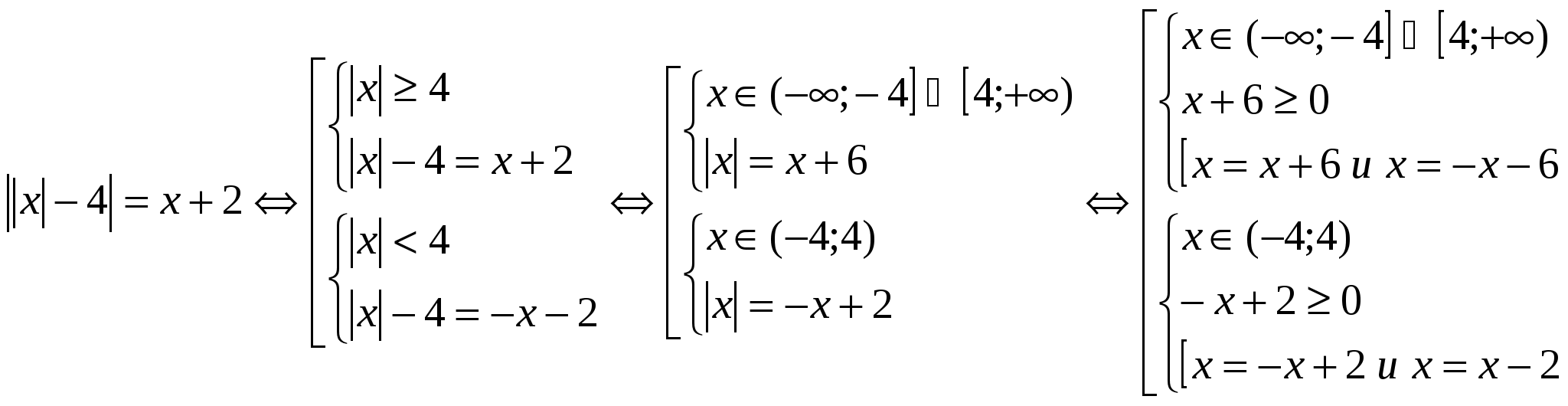
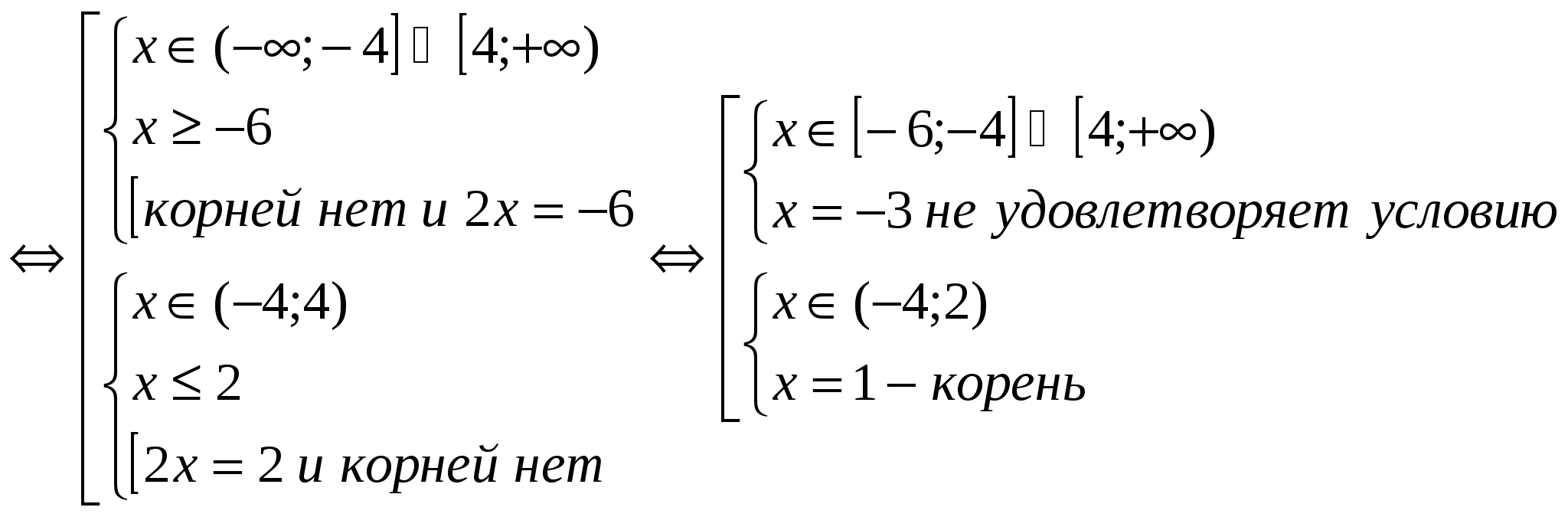(2)


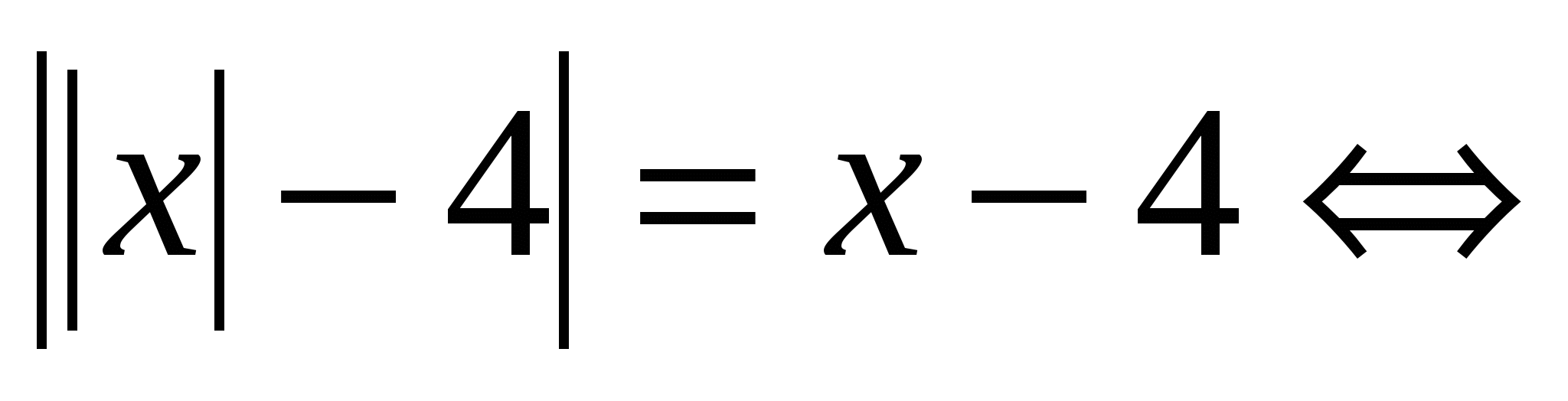
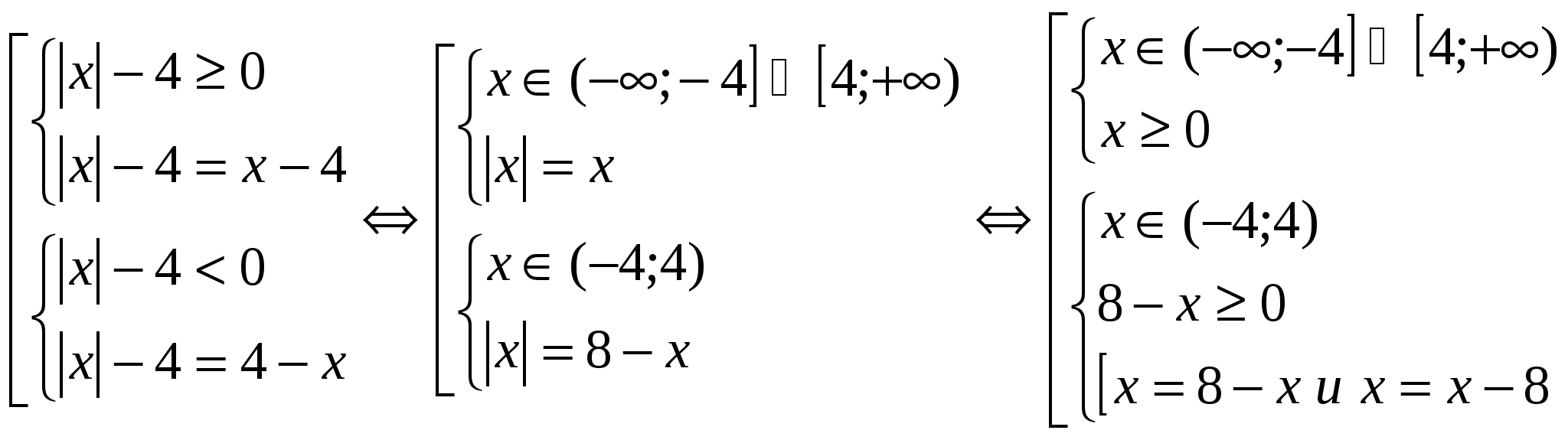
Answer:
Exercises: №36. Solve the equation, in the answer indicate the sum of the roots: 5 │3x-5│ \u003d 25 x №37. Solve the equation, if there are more than one roots, in the answer indicate the sum of the roots: │x + 2│ x - 3x - 10 = 1 №38. Solve the equation: 3 │2x -4│ \u003d 9 │x│ №39. Solve the equation, in the answer indicate the number of roots for: 2 │ sin x │ = √2 №40 . Solve the equation, in the answer indicate the number of roots:

Section 3. Logarithmic equations.

Before solving the following equations, it is necessary to review the properties of logarithms and the logarithmic function. Examples: №1. Solve the equation, in the answer indicate the product of the roots: log 2 (x + 1) 2 + log 2 │x + 1 │ \u003d 6 O.D.Z. x+1≠0 x≠ - 1

Case 1: if x ≥ - 1, then log 2 (x+1) 2 + log 2 (x+1) = 6 log 2 (x+1) 3 = log 2 2 6 (x+1) 3 = 2 6 x+1 = 4 x = 3 – satisfies the condition x ≥ - 1 2 case: if x log 2 (x+1) 2 + log 2 (-x-1) = 6 log 2 (x+1) 2 + log 2 (-(x+1)) = 6 log 2 (-(x+1) 3) = log 2 2 6- (x+1) 3 = 2 6- (x+1) = 4 x = - 5 – satisfies condition x - 1
Answer: The product of the roots is 15.
№2. Solve the equation, in the answer indicate the sum of the roots: lg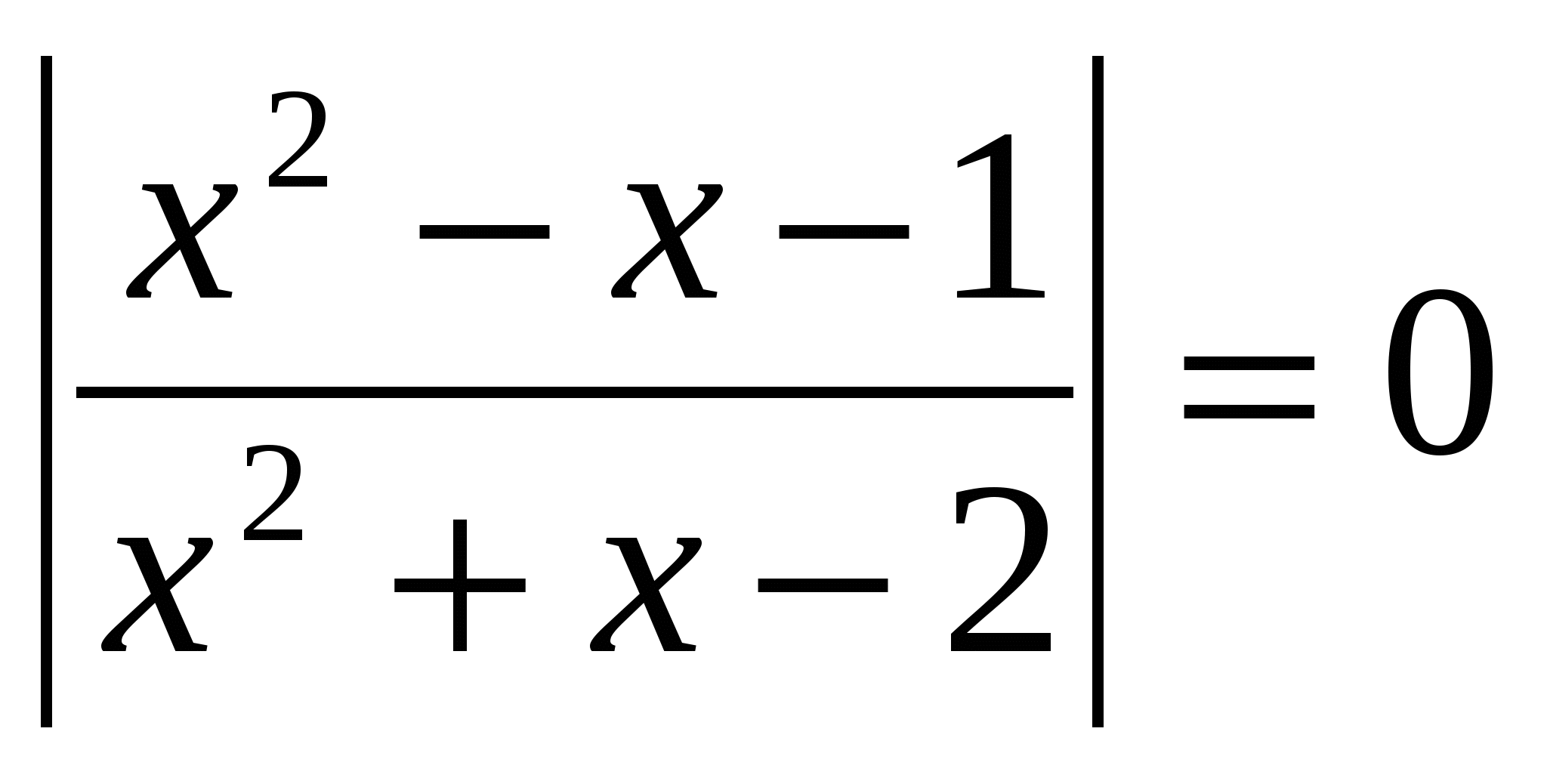
O.D.Z.

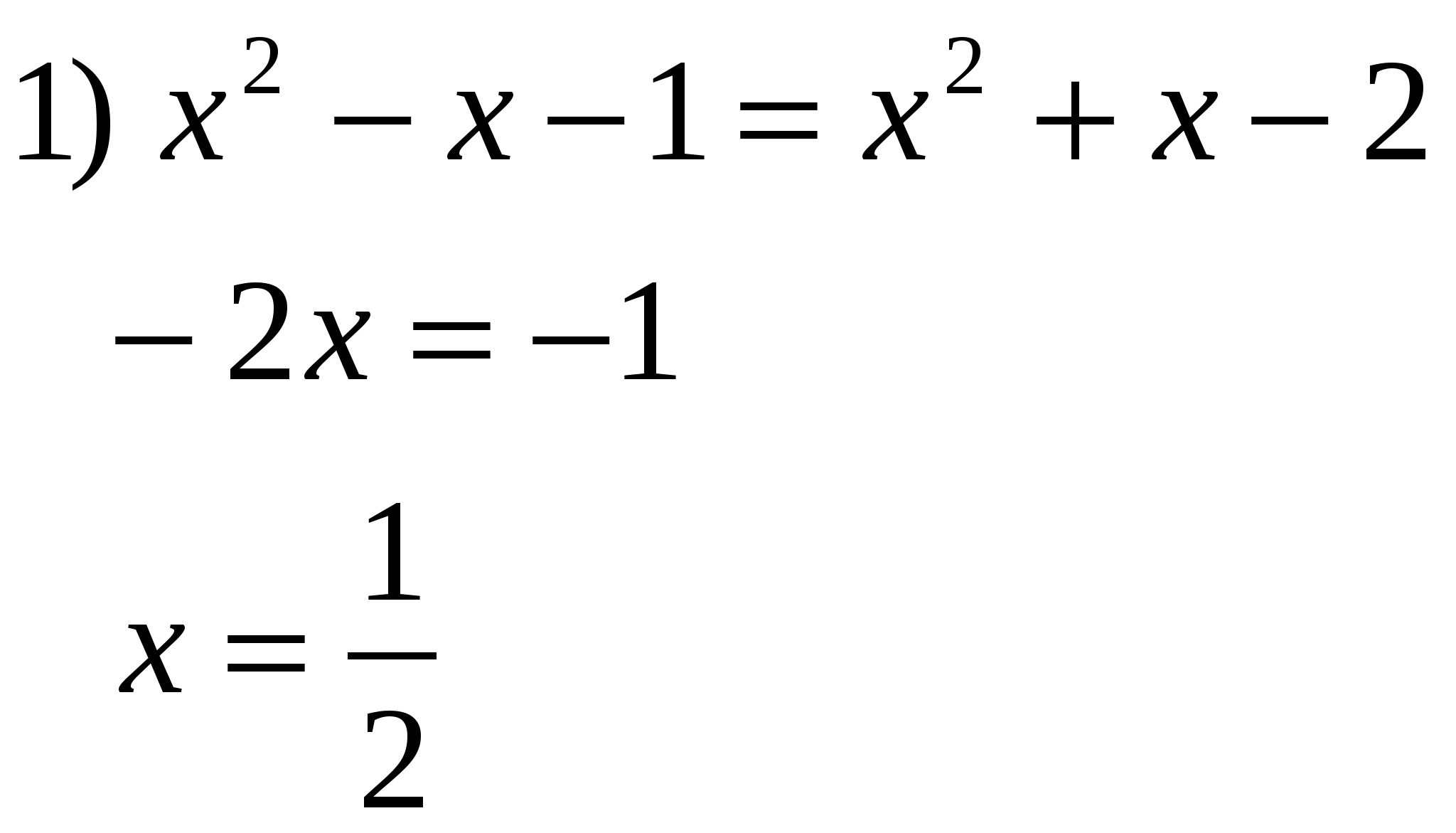
Answer: the sum of the roots is 0.5.
№3. Solve the equation: log 5
O.D.Z.

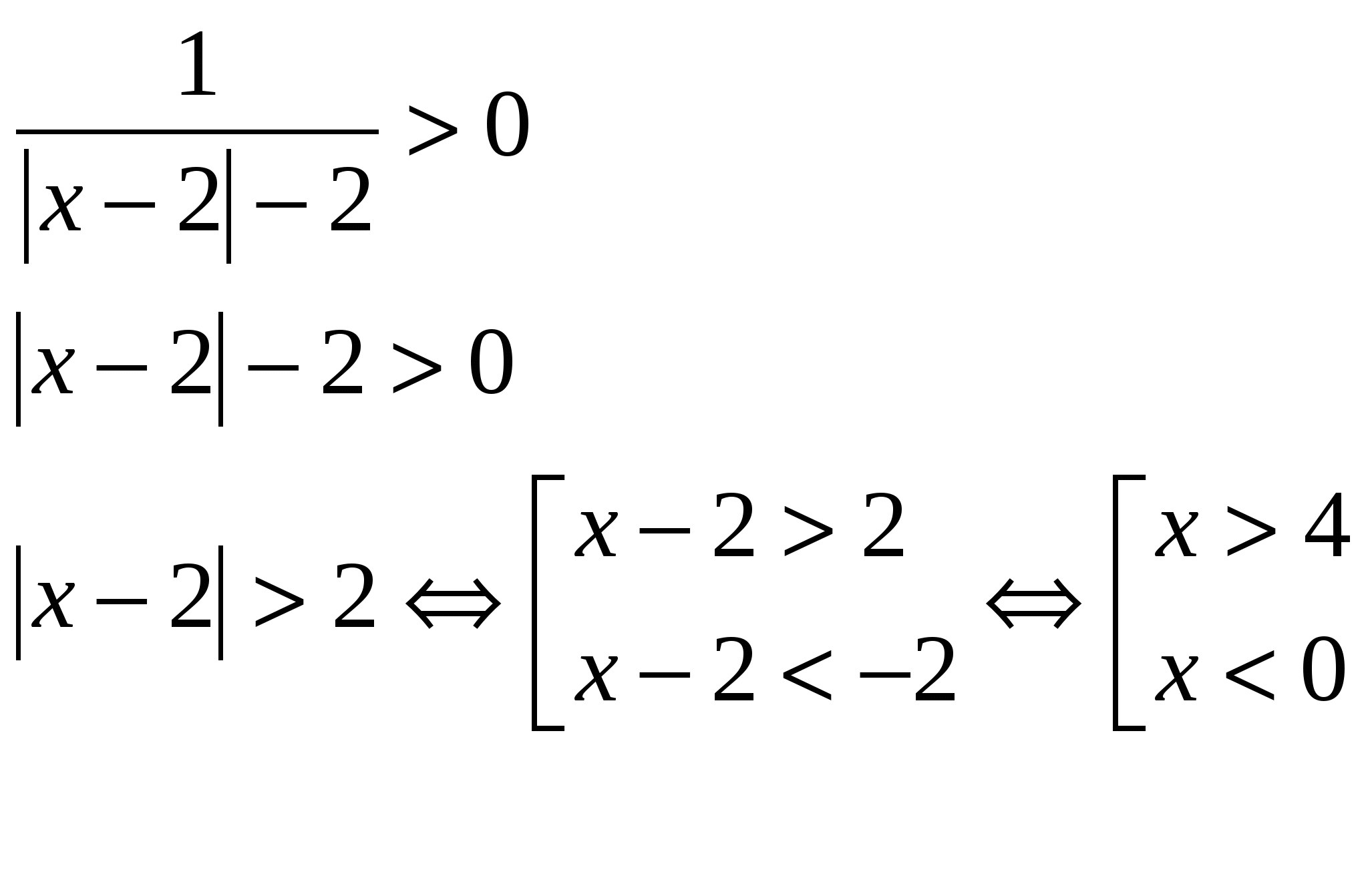
Answer: x = 9. №4. Solve the equation: │2 + log 0.2 x│+ 3 = │1 + log 5 x│ O.D.Z. x > 0 Let's use the formula for moving to another base. │2 - log 5 x│+ 3 = │1 + log 5 x│
│2 - log 5 x│- │1 + log 5 x│= - 3 Let's find the zeros of submodule expressions: x = 25; x \u003d These numbers divide the area of ​​\u200b\u200bpermissible values ​​into three intervals, so the equation is equivalent to the totality of three systems.
Answer: )