How to add fractions with different denominators. Subtraction of fractions with different denominators

Adding and subtracting fractions with the same denominators
Adding and subtracting fractions with different denominators
The concept of the NOC
Bringing fractions to the same denominator
How to add a whole number and a fraction

1 Adding and subtracting fractions with the same denominators

To add fractions with the same denominators, you need to add their numerators, and leave the denominator the same, for example:

To subtract fractions with the same denominators, subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator the same, for example:

To add mixed fractions, you must separately add their whole parts, and then add their fractional parts, and write the result as a mixed fraction,

If, when adding the fractional parts, an improper fraction is obtained, we select the integer part from it and add it to the integer part, for example:

2 Adding and subtracting fractions with different denominators

In order to add or subtract fractions with different denominators, you must first bring them to the same denominator, and then proceed as indicated at the beginning of this article. The common denominator of several fractions is the LCM (least common multiple). For the numerator of each of the fractions, additional factors are found by dividing the LCM by the denominator of this fraction. We'll look at an example later, after we figure out what an LCM is.

3 Least common multiple (LCM)

The least common multiple of two numbers (LCM) is the smallest natural number that is divisible by both of these numbers without a remainder. Sometimes the LCM can be found orally, but more often, especially when working with large numbers, you have to find the LCM in writing, using the following algorithm:

In order to find the LCM of several numbers, you need:

1. Decompose these numbers into prime factors
2. Take the largest expansion, and write these numbers as a product
3. Select in other expansions the numbers that do not occur in the largest expansion (or occur in it a smaller number of times), and add them to the product.
4. Multiply all the numbers in the product, this will be the LCM.

For example, let's find the LCM of numbers 28 and 21:

4Reducing fractions to the same denominator

Let's go back to adding fractions with different denominators.

When we reduce fractions to the same denominator, equal to the LCM of both denominators, we must multiply the numerators of these fractions by additional multipliers. You can find them by dividing the LCM by the denominator of the corresponding fraction, for example:

Thus, in order to bring fractions to one indicator, you must first find the LCM (that is, the smallest number that is divisible by both denominators) of the denominators of these fractions, then put additional factors on the numerators of the fractions. You can find them by dividing the common denominator (LCD) by the denominator of the corresponding fraction. Then you need to multiply the numerator of each fraction by an additional factor, and put the LCM as the denominator.

5How to add a whole number and a fraction

In order to add a whole number and a fraction, you just need to add this number in front of the fraction, and you get a mixed fraction, for example.

You can perform various actions with fractions, for example, adding fractions. Addition of fractions can be divided into several types. Each type of addition of fractions has its own rules and algorithm of actions. Let's take a closer look at each type of addition.

Adding fractions with the same denominators.

For example, let's see how to add fractions with a common denominator.

The hikers went on a hike from point A to point E. On the first day, they walked from point A to B, or \(\frac(1)(5)\) all the way. On the second day they went from point B to D or \(\frac(2)(5)\) the whole way. How far did they travel from the beginning of the journey to point D?

To find the distance from point A to point D, add the fractions \(\frac(1)(5) + \frac(2)(5)\).

Adding fractions with the same denominators is that you need to add the numerators of these fractions, and the denominator will remain the same.

\(\frac(1)(5) + \frac(2)(5) = \frac(1 + 2)(5) = \frac(3)(5)\)

In literal form, the sum of fractions with the same denominators will look like this:

\(\bf \frac(a)(c) + \frac(b)(c) = \frac(a + b)(c)\)

Answer: the tourists traveled \(\frac(3)(5)\) all the way.

Adding fractions with different denominators.

Consider an example:

Add two fractions \(\frac(3)(4)\) and \(\frac(2)(7)\).

To add fractions with different denominators, you must first find, and then use the rule for adding fractions with the same denominators.

For denominators 4 and 7, the common denominator is 28. The first fraction \(\frac(3)(4)\) must be multiplied by 7. The second fraction \(\frac(2)(7)\) must be multiplied by 4.

\(\frac(3)(4) + \frac(2)(7) = \frac(3 \times \color(red) (7) + 2 \times \color(red) (4))(4 \ times \color(red) (7)) = \frac(21 + 8)(28) = \frac(29)(28) = 1\frac(1)(28)\)

In literal form, we get the following formula:

\(\bf \frac(a)(b) + \frac(c)(d) = \frac(a \times d + c \times b)(b \times d)\)

Addition of mixed numbers or mixed fractions.

Addition occurs according to the law of addition.

For mixed fractions, add the integer parts to the integer parts and the fractional parts to the fractional parts.

If the fractional parts of mixed numbers have same denominators, then add the numerators, but the denominator remains the same.

Add mixed numbers \(3\frac(6)(11)\) and \(1\frac(3)(11)\).

\(3\frac(6)(11) + 1\frac(3)(11) = (\color(red) (3) + \color(blue) (\frac(6)(11))) + ( \color(red) (1) + \color(blue) (\frac(3)(11))) = (\color(red) (3) + \color(red) (1)) + (\color( blue) (\frac(6)(11)) + \color(blue) (\frac(3)(11))) = \color(red)(4) + (\color(blue) (\frac(6 + 3)(11))) = \color(red)(4) + \color(blue) (\frac(9)(11)) = \color(red)(4) \color(blue) (\frac (9)(11))\)

If the fractional parts of mixed numbers have different denominators, then we find a common denominator.

Let's add mixed numbers \(7\frac(1)(8)\) and \(2\frac(1)(6)\).

The denominator is different, so you need to find a common denominator, it is equal to 24. Multiply the first fraction \(7\frac(1)(8)\) by an additional factor of 3, and the second fraction \(2\frac(1)(6)\) on 4.

\(7\frac(1)(8) + 2\frac(1)(6) = 7\frac(1 \times \color(red) (3))(8 \times \color(red) (3) ) = 2\frac(1 \times \color(red) (4))(6 \times \color(red) (4)) =7\frac(3)(24) + 2\frac(4)(24 ) = 9\frac(7)(24)\)

Related questions:
How to add fractions?
Answer: first you need to decide what type the expression belongs to: fractions have the same denominators, different denominators or mixed fractions. Depending on the type of expression, we proceed to the solution algorithm.

How to solve fractions with different denominators?
Answer: you need to find a common denominator, and then follow the rule of adding fractions with the same denominators.

How to solve mixed fractions?
Answer: Add integer parts to integer parts and fractional parts to fractional parts.

Example #1:
Can the sum of two result in a proper fraction? Wrong fraction? Give examples.

\(\frac(2)(7) + \frac(3)(7) = \frac(2 + 3)(7) = \frac(5)(7)\)

The fraction \(\frac(5)(7)\) is a proper fraction, it is the result of the sum of two proper fractions \(\frac(2)(7)\) and \(\frac(3)(7)\).

\(\frac(2)(5) + \frac(8)(9) = \frac(2 \times 9 + 8 \times 5)(5 \times 9) =\frac(18 + 40)(45) = \frac(58)(45)\)

The fraction \(\frac(58)(45)\) is an improper fraction, it is the result of the sum of the proper fractions \(\frac(2)(5)\) and \(\frac(8)(9)\).

Answer: The answer is yes to both questions.

Example #2:
Add fractions: a) \(\frac(3)(11) + \frac(5)(11)\) b) \(\frac(1)(3) + \frac(2)(9)\).

a) \(\frac(3)(11) + \frac(5)(11) = \frac(3 + 5)(11) = \frac(8)(11)\)

b) \(\frac(1)(3) + \frac(2)(9) = \frac(1 \times \color(red) (3))(3 \times \color(red) (3)) + \frac(2)(9) = \frac(3)(9) + \frac(2)(9) = \frac(5)(9)\)

Example #3:
Write a mixed fraction as a sum natural number and a proper fraction: a) \(1\frac(9)(47)\) b) \(5\frac(1)(3)\)

a) \(1\frac(9)(47) = 1 + \frac(9)(47)\)

b) \(5\frac(1)(3) = 5 + \frac(1)(3)\)

Example #4:
Calculate the sum: a) \(8\frac(5)(7) + 2\frac(1)(7)\) b) \(2\frac(9)(13) + \frac(2)(13) \) c) \(7\frac(2)(5) + 3\frac(4)(15)\)

a) \(8\frac(5)(7) + 2\frac(1)(7) = (8 + 2) + (\frac(5)(7) + \frac(1)(7)) = 10 + \frac(6)(7) = 10\frac(6)(7)\)

b) \(2\frac(9)(13) + \frac(2)(13) = 2 + (\frac(9)(13) + \frac(2)(13)) = 2\frac(11 )(thirteen) \)

c) \(7\frac(2)(5) + 3\frac(4)(15) = 7\frac(2 \times 3)(5 \times 3) + 3\frac(4)(15) = 7\frac(6)(15) + 3\frac(4)(15) = (7 + 3)+(\frac(6)(15) + \frac(4)(15)) = 10 + \frac (10)(15) = 10\frac(10)(15) = 10\frac(2)(3)\)

Task #1:
At dinner they ate \(\frac(8)(11)\) of the cake, and in the evening at dinner they ate \(\frac(3)(11)\). Do you think the cake was completely eaten or not?

Solution:
The denominator of the fraction is 11, it indicates how many parts the cake was divided into. At lunch, we ate 8 pieces of cake out of 11. At dinner, we ate 3 pieces of cake out of 11. Let's add 8 + 3 = 11, we ate pieces of cake out of 11, that is, the whole cake.

\(\frac(8)(11) + \frac(3)(11) = \frac(11)(11) = 1\)

Answer: They ate the whole cake.

Lesson content

Adding fractions with the same denominators

Adding fractions is of two types:

1. Adding fractions with the same denominators
2. Adding fractions with different denominators

Let's start with adding fractions with the same denominators. Everything is simple here. To add fractions with the same denominators, you need to add their numerators, and leave the denominator unchanged. For example, let's add the fractions and . We add the numerators, and leave the denominator unchanged:

This example can be easily understood if we think of a pizza that is divided into four parts. If you add pizza to pizza, you get pizza:

Example 2 Add fractions and .

The answer is an improper fraction. If the end of the task comes, then it is customary to get rid of improper fractions. To get rid of an improper fraction, you need to select the whole part in it. In our case, the integer part is allocated easily - two divided by two is equal to one:

This example can be easily understood if we think of a pizza that is divided into two parts. If you add more pizzas to the pizza, you get one whole pizza:

Example 3. Add fractions and .

Again, add the numerators, and leave the denominator unchanged:

This example can be easily understood if we think of a pizza that is divided into three parts. If you add more pizzas to pizza, you get pizzas:

Example 4 Find the value of an expression

This example is solved in exactly the same way as the previous ones. The numerators must be added and the denominator left unchanged:

Let's try to depict our solution using a picture. If you add pizzas to a pizza and add more pizzas, you get 1 whole pizza and more pizzas.

As you can see, adding fractions with the same denominators is not difficult. It is enough to understand the following rules:

1. To add fractions with the same denominator, you need to add their numerators, and leave the denominator unchanged;

Adding fractions with different denominators

Now we will learn how to add fractions with different denominators. When adding fractions, the denominators of those fractions must be the same. But they are not always the same.

For example, fractions can be added because they have the same denominators.

But fractions cannot be added at once, because these fractions have different denominators. In such cases, fractions must be reduced to the same (common) denominator.

There are several ways to reduce fractions to the same denominator. Today we will consider only one of them, since the rest of the methods may seem complicated for a beginner.

The essence of this method lies in the fact that first (LCM) of the denominators of both fractions is sought. Then the LCM is divided by the denominator of the first fraction and the first additional factor is obtained. They do the same with the second fraction - the LCM is divided by the denominator of the second fraction and the second additional factor is obtained.

Then the numerators and denominators of the fractions are multiplied by their additional factors. As a result of these actions, fractions that had different denominators turn into fractions that have the same denominators. And we already know how to add such fractions.

Example 1. Add fractions and

First of all, we find the least common multiple of the denominators of both fractions. The denominator of the first fraction is the number 3, and the denominator of the second fraction is the number 2. The least common multiple of these numbers is 6

LCM (2 and 3) = 6

Now back to fractions and . First, we divide the LCM by the denominator of the first fraction and get the first additional factor. LCM is the number 6, and the denominator of the first fraction is the number 3. Divide 6 by 3, we get 2.

The resulting number 2 is the first additional factor. We write it down to the first fraction. To do this, we make a small oblique line above the fraction and write down the found additional factor above it:

We do the same with the second fraction. We divide the LCM by the denominator of the second fraction and get the second additional factor. LCM is the number 6, and the denominator of the second fraction is the number 2. Divide 6 by 2, we get 3.

The resulting number 3 is the second additional factor. We write it to the second fraction. Again, we make a small oblique line above the second fraction and write the found additional factor above it:

Now we are all set to add. It remains to multiply the numerators and denominators of fractions by their additional factors:

Look closely at what we have come to. We came to the conclusion that fractions that had different denominators turned into fractions that had the same denominators. And we already know how to add such fractions. Let's complete this example to the end:

Thus the example ends. To add it turns out.

Let's try to depict our solution using a picture. If you add pizzas to a pizza, you get one whole pizza and another sixth of a pizza:

Reduction of fractions to the same (common) denominator can also be depicted using a picture. Bringing the fractions and to a common denominator, we get the fractions and . These two fractions will be represented by the same slices of pizzas. The only difference will be that this time they will be divided into equal shares (reduced to the same denominator).

The first drawing shows a fraction (four pieces out of six) and the second picture shows a fraction (three pieces out of six). Putting these pieces together we get (seven pieces out of six). This fraction is incorrect, so we have highlighted the integer part in it. The result was (one whole pizza and another sixth pizza).

Note that we have painted this example in too much detail. V educational institutions it is not customary to write in such a detailed manner. You need to be able to quickly find the LCM of both denominators and additional factors to them, as well as quickly multiply the additional factors found by your numerators and denominators. While at school, we would have to write this example as follows:

But there is also back side medals. If at the first stages of studying mathematics you do not detailed records, then questions of the kind “Where does that number come from?”, “Why do fractions suddenly turn into completely different fractions? «.

To make it easier to add fractions with different denominators, you can use the following step-by-step instructions:

1. Find the LCM of the denominators of fractions;
2. Divide the LCM by the denominator of each fraction and get an additional multiplier for each fraction;
3. Multiply the numerators and denominators of fractions by their additional factors;
4. Add fractions that have the same denominators;
5. If the answer turned out to be an improper fraction, then select its whole part;

Example 2 Find the value of an expression .

Let's use the instructions above.

Step 1. Find the LCM of the denominators of fractions

Find the LCM of the denominators of both fractions. The denominators of the fractions are the numbers 2, 3 and 4

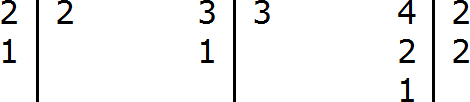
Step 2. Divide the LCM by the denominator of each fraction and get an additional multiplier for each fraction

Divide the LCM by the denominator of the first fraction. LCM is the number 12, and the denominator of the first fraction is the number 2. Divide 12 by 2, we get 6. We got the first additional factor 6. We write it over the first fraction:

Now we divide the LCM by the denominator of the second fraction. LCM is the number 12, and the denominator of the second fraction is the number 3. We divide 12 by 3, we get 4. We got the second additional factor 4. We write it over the second fraction:

Now we divide the LCM by the denominator of the third fraction. LCM is the number 12, and the denominator of the third fraction is the number 4. Divide 12 by 4, we get 3. We got the third additional factor 3. We write it over the third fraction:

Step 3. Multiply the numerators and denominators of fractions by your additional factors

We multiply the numerators and denominators by our additional factors:

Step 4. Add fractions that have the same denominators

We came to the conclusion that fractions that had different denominators turned into fractions that have the same (common) denominators. It remains to add these fractions. Add up:

The addition didn't fit on one line, so we moved the remaining expression to the next line. This is allowed in mathematics. When an expression does not fit on one line, it is carried over to the next line, and it is necessary to put an equal sign (=) at the end of the first line and at the beginning new line. The equal sign on the second line indicates that this is a continuation of the expression that was on the first line.

Step 5. If the answer turned out to be an improper fraction, then select the whole part in it

Our answer is an improper fraction. We must single out the whole part of it. We highlight:

Got an answer

Subtraction of fractions with the same denominators

There are two types of fraction subtraction:

1. Subtraction of fractions with the same denominators
2. Subtraction of fractions with different denominators

First, let's learn how to subtract fractions with the same denominators. Everything is simple here. To subtract another from one fraction, you need to subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator the same.

For example, let's find the value of the expression . To solve this example, it is necessary to subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator unchanged. Let's do this:

This example can be easily understood if we think of a pizza that is divided into four parts. If you cut pizzas from a pizza, you get pizzas:

Example 2 Find the value of the expression .

Again, from the numerator of the first fraction, subtract the numerator of the second fraction, and leave the denominator unchanged:

This example can be easily understood if we think of a pizza that is divided into three parts. If you cut pizzas from a pizza, you get pizzas:

Example 3 Find the value of an expression

This example is solved in exactly the same way as the previous ones. From the numerator of the first fraction, you need to subtract the numerators of the remaining fractions:

As you can see, there is nothing complicated in subtracting fractions with the same denominators. It is enough to understand the following rules:

1. To subtract another from one fraction, you need to subtract the numerator of the second fraction from the numerator of the first fraction, and leave the denominator unchanged;
2. If the answer turned out to be an improper fraction, then you need to select the whole part in it.

Subtraction of fractions with different denominators

For example, a fraction can be subtracted from a fraction, since these fractions have the same denominators. But a fraction cannot be subtracted from a fraction, since these fractions have different denominators. In such cases, fractions must be reduced to the same (common) denominator.

The common denominator is found according to the same principle that we used when adding fractions with different denominators. First of all, find the LCM of the denominators of both fractions. Then the LCM is divided by the denominator of the first fraction and the first additional factor is obtained, which is written over the first fraction. Similarly, the LCM is divided by the denominator of the second fraction and a second additional factor is obtained, which is written over the second fraction.

The fractions are then multiplied by their additional factors. As a result of these operations, fractions that had different denominators turn into fractions that have the same denominators. And we already know how to subtract such fractions.

Example 1 Find the value of an expression:

These fractions have different denominators, so you need to bring them to the same (common) denominator.

First, we find the LCM of the denominators of both fractions. The denominator of the first fraction is the number 3, and the denominator of the second fraction is the number 4. The least common multiple of these numbers is 12

LCM (3 and 4) = 12

Now back to fractions and

Let's find an additional factor for the first fraction. To do this, we divide the LCM by the denominator of the first fraction. LCM is the number 12, and the denominator of the first fraction is the number 3. Divide 12 by 3, we get 4. We write the four over the first fraction:

We do the same with the second fraction. We divide the LCM by the denominator of the second fraction. LCM is the number 12, and the denominator of the second fraction is the number 4. Divide 12 by 4, we get 3. We write a triple over the second fraction:

Now we are all set for subtraction. It remains to multiply the fractions by their additional factors:

We came to the conclusion that fractions that had different denominators turned into fractions that had the same denominators. And we already know how to subtract such fractions. Let's complete this example to the end:

Got an answer

Let's try to depict our solution using a picture. If you cut pizzas from a pizza, you get pizzas.

This is the detailed version of the solution. Being at school, we would have to solve this example in a shorter way. Such a solution would look like this:

Reduction of fractions and to a common denominator can also be depicted using a picture. Bringing these fractions to a common denominator, we get the fractions and . These fractions will be represented by the same pizza slices, but this time they will be divided into the same fractions (reduced to the same denominator):

The first drawing shows a fraction (eight pieces out of twelve), and the second picture shows a fraction (three pieces out of twelve). By cutting off three pieces from eight pieces, we get five pieces out of twelve. The fraction describes these five pieces.

Example 2 Find the value of an expression

These fractions have different denominators, so you first need to bring them to the same (common) denominator.

Find the LCM of the denominators of these fractions.

The denominators of the fractions are the numbers 10, 3 and 5. The least common multiple of these numbers is 30

LCM(10, 3, 5) = 30

Now we find additional factors for each fraction. To do this, we divide the LCM by the denominator of each fraction.

Let's find an additional factor for the first fraction. LCM is the number 30, and the denominator of the first fraction is the number 10. Divide 30 by 10, we get the first additional factor 3. We write it over the first fraction:

Now we find an additional factor for the second fraction. Divide the LCM by the denominator of the second fraction. LCM is the number 30, and the denominator of the second fraction is the number 3. Divide 30 by 3, we get the second additional factor 10. We write it over the second fraction:

Now we find an additional factor for the third fraction. Divide the LCM by the denominator of the third fraction. LCM is the number 30, and the denominator of the third fraction is the number 5. Divide 30 by 5, we get the third additional factor 6. We write it over the third fraction:

Now everything is ready for subtraction. It remains to multiply the fractions by their additional factors:

We came to the conclusion that fractions that had different denominators turned into fractions that have the same (common) denominators. And we already know how to subtract such fractions. Let's finish this example.

The continuation of the example will not fit on one line, so we move the continuation to the next line. Don't forget about the equal sign (=) on the new line:

The answer turned out to be a correct fraction, and everything seems to suit us, but it is too cumbersome and ugly. We should make it easier. What can be done? You can reduce this fraction.

To reduce a fraction, you need to divide its numerator and denominator by (gcd) the numbers 20 and 30.

So, we find the GCD of the numbers 20 and 30:

Now we return to our example and divide the numerator and denominator of the fraction by the found GCD, that is, by 10

Got an answer

Multiplying a fraction by a number

To multiply a fraction by a number, you need to multiply the numerator of the given fraction by this number, and leave the denominator the same.

Example 1. Multiply the fraction by the number 1.

Multiply the numerator of the fraction by the number 1

The entry can be understood as taking half 1 time. For example, if you take pizza 1 time, you get pizza

From the laws of multiplication, we know that if the multiplicand and the multiplier are interchanged, then the product will not change. If the expression is written as , then the product will still be equal to . Again, the rule for multiplying an integer and a fraction works:

This entry can be understood as taking half of the unit. For example, if there is 1 whole pizza and we take half of it, then we will have pizza:

Example 2. Find the value of an expression

Multiply the numerator of the fraction by 4

The answer is an improper fraction. Let's take a whole part of it:

The expression can be understood as taking two quarters 4 times. For example, if you take pizzas 4 times, you get two whole pizzas.

And if we swap the multiplicand and the multiplier in places, we get the expression. It will also be equal to 2. This expression can be understood as taking two pizzas from four whole pizzas:

Multiplication of fractions

To multiply fractions, you need to multiply their numerators and denominators. If the answer is an improper fraction, you need to select the whole part in it.

Example 1 Find the value of the expression .

Got an answer. It is desirable to reduce this fraction. The fraction can be reduced by 2. Then the final solution will take the following form:

The expression can be understood as taking a pizza from half a pizza. Let's say we have half a pizza:

How to take two-thirds from this half? First you need to divide this half into three equal parts:

And take two from these three pieces:

We'll get pizza. Remember what a pizza looks like divided into three parts:

One slice from this pizza and the two slices we took will have the same dimensions:

In other words, we are talking about the same pizza size. Therefore, the value of the expression is

Example 2. Find the value of an expression

Multiply the numerator of the first fraction by the numerator of the second fraction, and the denominator of the first fraction by the denominator of the second fraction:

The answer is an improper fraction. Let's take a whole part of it:

Example 3 Find the value of an expression

Multiply the numerator of the first fraction by the numerator of the second fraction, and the denominator of the first fraction by the denominator of the second fraction:

The answer turned out to be a correct fraction, but it will be good if it is reduced. To reduce this fraction, you need to divide the numerator and denominator of this fraction by the largest common divisor(gcd) numbers 105 and 450.

So, let's find the GCD of the numbers 105 and 450:

Now we divide the numerator and denominator of our answer to the GCD that we have now found, that is, by 15

Representing an integer as a fraction

Any whole number can be represented as a fraction. For example, the number 5 can be represented as . From this, the five will not change its meaning, since the expression means “the number five divided by one”, and this, as you know, is equal to five:

Reverse numbers

Now we will get acquainted with interesting topic in mathematics. It's called "reverse numbers".

Definition. Reverse to numbera is the number that, when multiplied bya gives a unit.

Let's substitute in this definition instead of a variable a number 5 and try to read the definition:

Reverse to number 5 is the number that, when multiplied by 5 gives a unit.

Is it possible to find a number that, when multiplied by 5, gives one? It turns out you can. Let's represent five as a fraction:

Then multiply this fraction by itself, just swap the numerator and denominator. In other words, let's multiply the fraction by itself, only inverted:

What will be the result of this? If we continue to solve this example, we get one:

This means that the inverse of the number 5 is the number, since when 5 is multiplied by one, one is obtained.

The reciprocal can also be found for any other integer.

You can also find the reciprocal for any other fraction. To do this, it is enough to turn it over.

Division of a fraction by a number

Let's say we have half a pizza:

Let's divide it equally between two. How many pizzas will each get?

It can be seen that after splitting half of the pizza, two equal pieces were obtained, each of which makes up a pizza. So everyone gets a pizza.

Division of fractions is done using reciprocals. Reciprocals allow you to replace division with multiplication.

To divide a fraction by a number, you need to multiply this fraction by the reciprocal of the divisor.

Using this rule, we will write down the division of our half of the pizza into two parts.

So, you need to divide the fraction by the number 2. Here the dividend is a fraction and the divisor is 2.

To divide a fraction by the number 2, you need to multiply this fraction by the reciprocal of the divisor 2. The reciprocal of the divisor 2 is a fraction. So you need to multiply by

Fractional expressions are difficult for a child to understand. Most people have difficulties with . When studying the topic "addition of fractions with integers", the child falls into a stupor, finding it difficult to solve the task. In many examples, a series of calculations must be performed before an action can be performed. For example, convert fractions or convert an improper fraction to a proper one.

Explain to the child clearly. Take three apples, two of which will be whole, and the third will be cut into 4 parts. Separate one slice from the cut apple, and put the remaining three next to two whole fruits. We get ¼ apples on one side and 2 ¾ on the other. If we combine them, we get three whole apples. Let's try to reduce 2 ¾ apples by ¼, that is, remove one more slice, we get 2 2/4 apples.

Let's take a closer look at actions with fractions, which include integers:

First, let's recall the calculation rule for fractional expressions with a common denominator:

At first glance, everything is easy and simple. But this applies only to expressions that do not require conversion.

How to find the value of an expression where the denominators are different

In some tasks, it is necessary to find the value of an expression where the denominators are different. Consider a specific case:
3 2/7+6 1/3

Find the value of this expression, for this we find a common denominator for two fractions.

For the numbers 7 and 3, this is 21. We leave the integer parts the same, and reduce the fractional parts to 21, for this we multiply the first fraction by 3, the second by 7, we get:
6/21+7/21, do not forget that whole parts are not subject to conversion. As a result, we get two fractions with one denominator and calculate their sum:
3 6/21+6 7/21=9 15/21
What if the result of addition is an improper fraction that already has an integer part:
2 1/3+3 2/3
In this case, we add the integer parts and fractional parts, we get:
5 3/3, as you know, 3/3 is one, so 2 1/3+3 2/3=5 3/3=5+1=6

With finding the sum, everything is clear, let's analyze the subtraction:

From all that has been said, the rule of operations on mixed numbers follows, which sounds like this:

• If it is necessary to subtract an integer from a fractional expression, it is not necessary to represent the second number as a fraction, it is enough to operate only on integer parts.

Let's try to calculate the value of expressions on our own:

Let's take a closer look at the example under the letter "m":

4 5/11-2 8/11, the numerator of the first fraction is less than the second. To do this, we take one integer from the first fraction, we get,
3 5/11+11/11=3 whole 16/11, subtract the second from the first fraction:
3 16/11-2 8/11=1 whole 8/11

• Be careful when completing the task, do not forget to convert improper fractions to mixed ones, highlighting the whole part. To do this, it is necessary to divide the value of the numerator by the value of the denominator, what happened, takes the place of the integer part, the remainder will be the numerator, for example:

19/4=4 ¾, check: 4*4+3=19, in the denominator 4 remains unchanged.

Summarize:

Before proceeding with the task related to fractions, it is necessary to analyze what kind of expression it is, what transformations need to be performed on the fraction in order for the solution to be correct. Look for more rational solutions. Don't go the hard way. Plan all the actions, decide first in a draft version, then transfer to a school notebook.

To avoid confusion when solving fractional expressions, it is necessary to follow the sequence rule. Decide everything carefully, without rushing.

The rules for adding fractions with different denominators are very simple.

Consider the rules for adding fractions with different denominators in steps:

1. Find the LCM (least common multiple) of the denominators. The resulting LCM will be the common denominator of the fractions;

2. Bring fractions to a common denominator;

3. Add fractions reduced to a common denominator.

On the simple example Learn how to add fractions with different denominators.

Example

An example of adding fractions with different denominators.

Add fractions with different denominators:

1	+	5
6		12

Let's decide step by step.

1. Find the LCM (least common multiple) of the denominators.

The number 12 is divisible by 6.

From this we conclude that 12 is the least common multiple of the numbers 6 and 12.

Answer: the nok of the numbers 6 and 12 is 12:

LCM(6, 12) = 12

The resulting NOC will be the common denominator of the two fractions 1/6 and 5/12.

2. Bring fractions to a common denominator.

In our example, only the first fraction needs to be reduced to a common denominator of 12, because the second fraction already has a denominator of 12.

Divide the common denominator of 12 by the denominator of the first fraction:

2 has an additional multiplier.

Multiply the numerator and denominator of the first fraction (1/6) by an additional factor of 2.